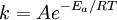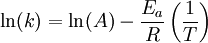Arrhenius plot

An Arrhenius plot displays the logarithm of a rate (ln(k), ordinate axis) plotted against inverse temperature (1 / T, abscissa). Arrhenius plots are often used to analyze the effect of temperature on the rates of chemical reactions. For a single rate-limited thermally activated process, an Arrhenius plot gives a straight line, from which the activation energy and the pre-exponential factor can both be determined.

Additional recommended knowledge

How to quickly check pipettes?

Don't let static charges disrupt your weighing accuracy

Correct Test Weight Handling Guide: 12 Practical Tips

The Arrhenius equation given in the form:

can be written equivalently as:

When plotted in the manner described above, the value of the extrapolated "y-intercept" will correspond to ln(A), and the gradient of the line will be equal to − E_a / R.

The pre-exponential factor, A, is a constant of proportionality that takes into account a number of factors such as the frequency of collision between and the orientation of the reacting particles.

The expression − E_a / RT represents the fraction of the molecules present in a gas which have energies equal to or in excess of activation energy at a particular temperature.

See also

• Arrhenius equation