To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Solenoidal vector fieldIn vector calculus a solenoidal vector field is a vector field v with divergence zero: Product highlightThis condition is satisfied whenever v has a vector potential, because if then The converse also holds: for any solenoidal v there exists a vector potential A such that The divergence theorem, gives the equivalent integral definition of a solenoidal field; namely that for any closed surface S, the net total flux through the surface must be zero:
where Examples
|
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Solenoidal_vector_field". A list of authors is available in Wikipedia. |


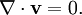




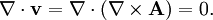
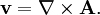 (Strictly speaking, this holds only subject to certain technical conditions on v, see Helmholtz decomposition.)
(Strictly speaking, this holds only subject to certain technical conditions on v, see Helmholtz decomposition.)
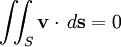 ,
,
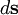 is the outward normal to each surface element.
is the outward normal to each surface element.


