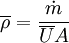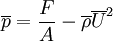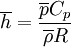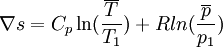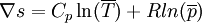To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Stream thrust averagingStream thrust averaging is a process used to convert 3 dimensional flow through a duct into 1 dimensional uniform flow. It makes the assumptions that the flow is mixed adiabatically and without friction. However, due to the mixing process, there is a net increase in the entropy of the system. Although there is an increase in entropy, the stream thrust averaged values are more representative of the flow than a simple average as a simple average would violate the 2nd Law of Thermodynamics. Product highlightThe following equations apply to a perfect gas. Stream Thrust Mass Flow Stagnation Enthalpy Solving for
2nd Law of Thermodynamics The values T1 and p1 are unknown and may be dropped from the formulation. The value of entropy is not necessary, only that the value is positive. One possible unreal solution for the stream thrust averaged velocity yields a negative entropy. Another method of determining the proper solution is to take a simple average of the velocity and determining which value is closer to the stream thrust averaged velocity. References
|
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Stream_thrust_averaging". A list of authors is available in Wikipedia. |





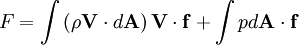

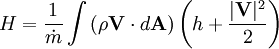
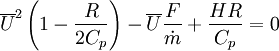
 yields 2 solutions. They must both be analyzed to determine which is the physical solution. One will usually be a subsonic root and the other a supersonic root. If it is not clear which value of Velocity is correct, the 2nd Law of Thermodynamics may be applied.
yields 2 solutions. They must both be analyzed to determine which is the physical solution. One will usually be a subsonic root and the other a supersonic root. If it is not clear which value of Velocity is correct, the 2nd Law of Thermodynamics may be applied.