My watch list
my.chemeurope.com  

my.chemeurope.com

With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.

  • My watch list
  • My saved searches
  • My saved topics
  • My newsletter
Register free of charge
Login  
eng  
DeutschEnglishFrançaisEspañol

Canonical probability distribution



In thermal physics, the canonical probability distribution is a statistical function which equates to the Boltzmann factor divided by the partition function. The function was introduced by Willard Gibbs in his 1901 Elementary Principles in Statistical Mechanics.

The probability of an element of the canonical ensemble having energy Ei is given by: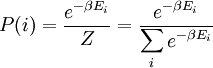

P(i)=\frac{e^{-\beta E_i}}{Z}=\frac{e^{-\beta E_i}}{\displaystyle\sum_{i} e^{-\beta E_i}}

Where \beta = \frac{1}{k_B T} and kB is the Boltzmann Constant.

 
This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Canonical_probability_distribution". A list of authors is available in Wikipedia.
Your browser is not current. Microsoft Internet Explorer 6.0 does not support some functions on Chemie.DE