Helicoid

The helicoid, after the plane and the catenoid, is the third minimal surface to be known. It was first discovered by Jean Baptiste Meusnier in 1776. Its name derives from its similarity to the helix: for every point on the helicoid there is a helix contained in the helicoid which passes through that point.

Additional recommended knowledge

What is the Sensitivity of my Balance?

Weighing the right way

How to quickly check pipettes?

The helicoid is also a ruled surface, meaning that it is a trace of a line. Alternatively, for any point on the surface, there is a line on the surface passing through it.

The helicoid and the catenoid are parts of a family of helicoid-catenoid minimal surfaces.

The helicoid is shaped like the Archimedes' screw, but extends infinitely in all directions. It can be described by the following parametric equations in Cartesian coordinates:

where ρ and θ range from negative infinity to positive infinity, while α is a constant. If α is positive then the helicoid is right-handed as shown in the figure; if negative then left handed.

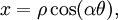
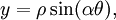
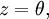
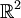The helicoid is homeomorphic to the plane . To see this, let alpha decrease continuously from its given value down to zero. Each intermediate value of α will describe a different helicoid, until α = 0 is reached and the helicoid becomes a vertical plane.

Conversely, a plane can be turned into a helicoid by choosing a line, or axis, on the plane then twisting the plane around that axis.