To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
K-space (MRI)Template:DISPLAYTITLE:k-space (MRI) Product highlightk-space is a formalism widely used in magnetic resonance imaging independently introduced in 1983 by Ljunggren[1] and Twieg[2]. Simply speaking, k-space is the temporary image space in which data from digitized MR signals are stored during data acquisition. When k-space is full (at the end of the scan), the data are mathematically processed to produce a final image. Thus k-space holds raw data before reconstruction. k-space is in spatial frequency domain. Thus if we define kFE and kPE such that and where FE refers to frequency encoding, PE to phase encoding, Δt is the sampling time (the reciprocal of sampling frequency), τ is the duration of GPE, k-space has the same number of rows and columns as the final image. During the scan, k-space is filled with raw data one line per TR. Although a strict mathematical proof does not exist and counterexamples can be provided, in most cases it is safe to say that data in the middle of k-space contain the signal to noise and contrast information for the image, while data around the outside of the image contain all the information about the image resolution. This is the basis for advanced scanning techniques, such as the keyhole acquisition, in which a first complete k-space is acquired, and subsequent scans are performed by acquiring just the central part of the k-space; in this way, different contrast images can be acquired without the need of running full scans. A nice symmetry property exist in the k-space, descending from the fact that the object imaged is a contrast-weighted proton density and thus a real quantity, relating the signal at two opposite locations in k-space: where the star denotes complex conjugation. Thus k-space information is somewhat redundant, and an image can be reconstructed using only one half of the k-space, either in the PE direction saving scan time (such a technique is known as half Fourier or half scan) or in the FE direction, allowing for lower sampling frequencies and/or shorter echo times (such a technique is known as half echo). References
Further Reading
|
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "K-space_(MRI)". A list of authors is available in Wikipedia. |





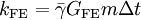
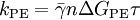
 (gamma bar) is the
(gamma bar) is the 


