To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Rarita-Schwinger equationIn theoretical physics, the Rarita-Schwinger equation is the relativistic field equation of spin-3/2 fermions. It is similar to the Dirac equation for spin-1/2 fermions. This equation was first introduced by William Rarita and Julian Schwinger in 1941. In modern notation it can be written as: Product highlightwhere εμνρσ is the Levi-Civita symbol, γ5 and γν are Dirac matrices, m is the mass and ψμ is a vector-valued spinor with additional components compared to the four component spinor in the Dirac equation. It corresponds to the where the bar above ψμ denotes the Dirac adjoint. As in the case of the Dirac equation, electromagnetic interaction can be added simply by promoting the partial derivative to gauge covariant derivative: The massless Rarita-Schwinger equation has a gauge symmetry, under the gauge transformation of "Weyl" and "Majorana" versions of the Rarita-Schwinger equation also exist. This equation is useful for the wave function of composite objects like Delta (Δ) baryons or for proposed elementary fields like the gravitino. So far, no fundamental particle with spin 3/2 has been found experimentally. Drawbacks of the formalism
The current description of massive, higher spin fields through either Rarita-Schwinger or Fierz–Pauli formalisms is afflicted with several maladies. Upon gauging, high spin fields suffer from acausal, superluminal propagation; besides, the quantization of these systems in interaction with electromagnetism is essentially flawed. Also, algebraic inconsistencies appear upon gauging which can only be avoided by requiring that all equations involving derivatives be obtainable from a Lagrangian, a procedure that becomes involved because of the need of introducing auxiliary fields in order to obtain all constraints from the Lagrangian. In 1969, Velo and Zwanziger showed that the Rarita–Schwinger lagrangian coupled to electromagnetism leads to equation with solutions representing wavefronts, some of which propagate faster than light. There are some Lorentz frames that allow the consistent formulation of quantum mechanics and quantum field theory; there are some others that don’t. References
|
||
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Rarita-Schwinger_equation". A list of authors is available in Wikipedia. |


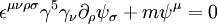



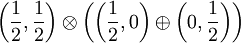 representation of the Lorentz group, or rather, its
representation of the Lorentz group, or rather, its 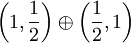 part.
This field equation can be derived from the following Lagrangian:
part.
This field equation can be derived from the following Lagrangian:
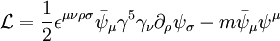

 , where
, where 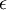 is an arbitrary spinor field.
is an arbitrary spinor field.


