To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Helicity (fluid mechanics)
In fluid mechanics, helicity is the extent to which corkscrew-like motion occurs. If a parcel of fluid is moving, undergoing solid body motion rotating about an axis parallel to the direction of motion, it will have helicity. If the rotation is clockwise when viewed from ahead of the body, the helicity will be positive, if counterclockwise, it will be negative. Product highlightFormally, helicity is defined as The concept is interesting because it is a conserved quantity: H is unchanged in a fluid obeying the Navier-Stokes equations for incompressible fluids. This is analogous to the conservation of magnetic helicity. Helicity is a useful concept in theoretical descriptions of turbulence. MeteorologyIn meteorology [1] , helicity correspond to the transfer of vorticity from the environment to an air parcel in convective motion. Here the definition of helicity is simplified to only use the horizontal component of wind and vorticity: According to this formula, if the horizontal wind does not change direction with altitude, H will be zero as the product of Vh and This notion is used to predict the possibility of tornadic development in a thundercloud. In this case, the vertical integration will be limited below cloud tops (generally 3 km or 10,000 feet) and the horizontal wind will be calculated to wind relative to the storm in subtracting its motion: Critical values of SRH (Storm Relative Helicity) for tornadic development, as researched in North America[2], are:
Helicity in itself is not the only component of severe thunderstorms and those values are to be taken with caution. That is why the Energy Helicity Index (EHI) has been created. It is the result of SRH multiplied by the CAPE (Convective Available Potential Energy) and then divided by a threshold CAPE . This incorporates not only the helicity but the energy of the air parcel and thus tries to eliminate weak potential for thunderstorms even in strong SRH regions. The critical values of EHI:
References
Bibliography
|
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Helicity_(fluid_mechanics)". A list of authors is available in Wikipedia. |





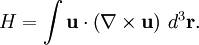
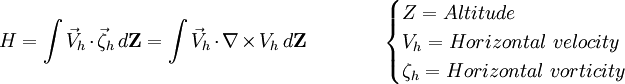
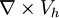 are perpendicular one to the other making their scalar product nil. H is then positive if the wind turns (clockwise) with altitude and negative if it backs (counter-clockwise). Helicity has energy units per units of mass (
are perpendicular one to the other making their scalar product nil. H is then positive if the wind turns (clockwise) with altitude and negative if it backs (counter-clockwise). Helicity has energy units per units of mass (


