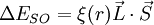To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Fine structureIn atomic physics, the fine structure describes the splitting of the spectral lines of atoms. The gross structure of line spectra is due to the principal quantum number n, giving the main electron shells of atoms. However, on closer examination, electron shells with n > 1 exhibit fine structure, and lines are split due to spin-orbit coupling (the energy difference between the electron spin being parallel or antiparallel to the electron's orbital moment). This gives rise to for example the doublet in the yellow sodium D-line. The fine structure of hydrogen is actually two separate corrections to the Bohr energies: one due to the relativistic motion of the electron, and the other due to spin-orbit coupling; see Lamb shift. Fine level structure can be split further by interaction with the magnetic moment of the nucleus (hyperfine structure). Product highlight
Scalar relativistic correctionClassically, the kinetic energy term of the Hamiltonian is: However, when considering special relativity, we must use a relativistic form of the kinetic energy, where the first term is the total relativistic energy, and the second term is the rest energy of the electron. Expanding this we find Then, the first order correction to the Hamiltonian is Using this as a perturbation, we can calculate the first order energy corrections due to relativistic effects. where ψ0 is the unperturbed wave function. Recalling the unperturbed Hamiltonian, we see We can use this result to further calculate the relativistic correction: For the hydrogen atom, Spin-orbit couplingThe spin-orbit correction arises when we shift from the standard frame of reference (where the electron orbits the nucleus) into one where the electron is stationary and the nucleus instead orbits it. In this case the orbiting nucleus functions as an effective current loop, which in turn will generate a magnetic field. However, the electron itself has a magnetic moment due to its intrinsic angular momentum. The two magnetic vectors, See alsoReferences
|
|
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Fine_structure". A list of authors is available in Wikipedia. |





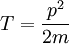
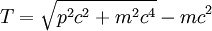
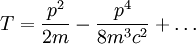
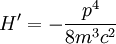
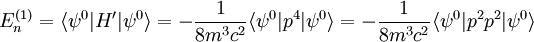
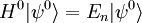
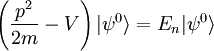
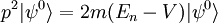
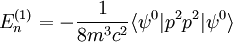
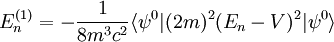
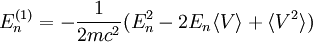
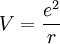 ,
, 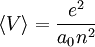 , and
, and 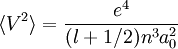 where
where 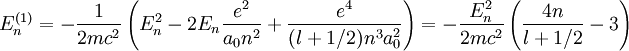
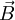 and
and 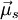 couple together so that there is a certain energy cost depending on their relative orientation. This gives rise to the energy correction of the form
couple together so that there is a certain energy cost depending on their relative orientation. This gives rise to the energy correction of the form