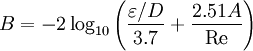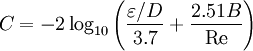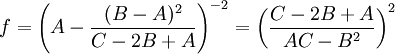My watch list
my.chemeurope.com  

my.chemeurope.com

With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.

  • My watch list
  • My saved searches
  • My saved topics
  • My newsletter
Register free of charge
Login  
eng  
DeutschEnglishFrançaisEspañol

Serghide's solution



 It has been suggested that this article or section be merged into Frictional pressure drop. (Discuss)

Serghide's solution is used to solve directly for the Darcy-Weisbach friction factor f for a full-flowing circular pipe. It is an approximation of the implicit Colebrook-White equation.

The solution involves calculating three intermediate values and then substituting those values into a final equation.

A = -2\log_{10}\left( {\varepsilon/D\over 3.7} + {12\over \mbox{Re}}\right)
B = -2\log_{10} \left({\varepsilon/D\over 3.7} + {2.51 A \over \mbox{Re}}\right)
C = -2\log_{10} \left({\varepsilon/D\over 3.7} + {2.51 B \over \mbox{Re}}\right)
f = \left(A - \frac{(B - A)^2}{C - 2B + A}\right)^{-2} = \left(\frac {C - 2B + A} {AC - B^2}\right)^2

where f is a function of:

  • roughness height, ε (m, ft)
  • pipe diameter, D (m, ft)
  • Reynolds number, Re (unitless).

The equation was found to match the Colebrook-White equation within 0.0031% for all practical applications.

References

  • http://www.cheresources.com/colebrook2.shtml
 
This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Serghide's_solution". A list of authors is available in Wikipedia.
Your browser is not current. Microsoft Internet Explorer 6.0 does not support some functions on Chemie.DE