To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Zeeman effectThe Zeeman effect (pronounced /ˈzeɪmɑːn/) is the splitting of a spectral line into several components in the presence of a static magnetic field. It is analogous to the Stark effect, the splitting of a spectral line into several components in the presence of an electric field. The Zeeman effect is very important in applications such as nuclear magnetic resonance spectroscopy, electron spin resonance spectroscopy, magnetic resonance imaging (MRI) and Mössbauer spectroscopy. When the spectral lines are absorption lines, the effect is called Inverse Zeeman effect. The Zeeman effect is named after the Dutch physicist Pieter Zeeman. Product highlight
IntroductionIn most atoms, there exist several electronic configurations that have the same energy, so that transitions between different pairs of configurations correspond to a single spectral line. The presence of a magnetic field breaks the degeneracy, since it interacts in a different way with electrons with different quantum numbers, slightly modifying their energies. The result is that, where there were several configurations with the same energy, now there are different energies, which give rise to several very close spectral lines.
Without a magnetic field, configurations a, b and c have the same energy, as do d, e and f. The presence of a magnetic field splits the energy levels. A line produced by a transition from a, b or c to d, e or f now will be several lines between different combinations of a, b, c and d, e, f. Not all transitions will be possible, as regulated by the transition rules. Since the distance between the Zeeman sub-levels is proportional to the magnetic field, this effect is used by astronomers to measure the magnetic field of the Sun and other stars. There is also an anomalous Zeeman effect that appears on transitions where the net spin of the electrons is not 0, the number of Zeeman sub-levels being even instead of odd if there's an uneven number of electrons involved. It was called "anomalous" because the electron spin had not yet been discovered, and so there was no good explanation for it at the time that Zeeman observed the effect. If the magnetic field strength is too high, the effect is no longer linear; at even higher field strength, electron coupling is disturbed and the spectral lines rearrange. This is called the Paschen-Back effect. Theoretical presentationThe total Hamiltonian of an atom in a magnetic field is H = H0 + HM, where H0 is the unperturbed Hamiltonian of the atom, and HM is perturbation due to the magnetic field:
where
where μB is the Bohr magneton,
where gl = 1 or
where If the interaction term VM is small (less than the fine structure), it can be treated as a perturbation; this is the Zeeman effect proper. In the Paschen-Back effect, described below, VM exceeds the LS coupling significantly (but is still small compared to H0). In ultrastrong magnetic fields, the magnetic-field interaction may exceed H0, in which case the atom can no longer exist in its normal meaning, and one talks about Landau levels instead. There are, of course, intermediate cases which are more complex than these limit cases. Weak field (Zeeman effect)If the spin-orbit interaction dominates over the effect of the external magnetic field,
and for the (time-)"averaged" orbital vector:
Thus,
Using
and:
using Combining everything and taking
where the quantity in square brackets is the Lande g-factor gJ of the atom (gL = 1 and Example: Lyman alpha transition in hydrogenThe Lyman alpha transition in hydrogen in the presence of the spin-orbit interaction involves the transitions
In the presence of an external magnetic field, the weak-field Zeeman effect splits the 1S1/2 and 2P1/2 states into 2 levels each (mj = 1 / 2, − 1 / 2) and the 2P3/2 state into 4 levels (mj = 3 / 2,1 / 2, − 1 / 2, − 3 / 2). The Lande g-factors for the three levels are:
Note in particular that the size of the energy splitting is different for the different orbitals, because the gJ values are different.
Strong field (Paschen-Back effect)When the magnetic-field perturbation significantly exceeds the spin-orbit interaction, one can safely assume [H0,S] = 0. This allows the expectation values of Lz and Sz to be easily evaluated for a state
The above may be read as implying that the LS-coupling is completely broken by the external field. The ml and ms are still "good" quantum numbers. Together with the selection rules for an electric dipole transition, i.e., See also
ReferencesHistorical
Modern
Categories: Atomic physics | Foundational quantum physics |
|
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Zeeman_effect". A list of authors is available in Wikipedia. |





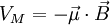 ,
,
 is the
is the 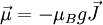 ,
,
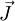 is the total electronic angular momentum, and
is the total electronic angular momentum, and 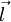 and the spin angular momentum
and the spin angular momentum 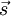 , with each multiplied by the appropriate
, with each multiplied by the appropriate 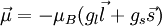 ,
,
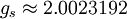 (the latter is called the anomalous gyromagnetic ratio; the deviation of the value from 2 is due to the relativistic effects). In the case of the
(the latter is called the anomalous gyromagnetic ratio; the deviation of the value from 2 is due to the relativistic effects). In the case of the 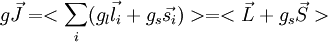 ,
,
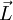 and
and 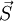 are the total orbital momentum and spin of the atom, and averaging is done over a state with a given value of the total angular momentum.
are the total orbital momentum and spin of the atom, and averaging is done over a state with a given value of the total angular momentum.
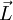 and
and 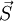 are not separately conserved, only the total angular momentum
are not separately conserved, only the total angular momentum 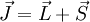 is. The spin and orbital angular momentum vectors can be thought of as precessing about the (fixed) total angular momentum vector
is. The spin and orbital angular momentum vectors can be thought of as precessing about the (fixed) total angular momentum vector 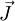 . The (time-)"averaged" spin vector is then the projection of the spin onto the direction of
. The (time-)"averaged" spin vector is then the projection of the spin onto the direction of 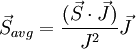 .
.
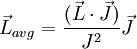 .
.
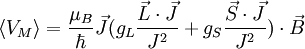 .
.
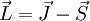 and squaring both sides, we get
and squaring both sides, we get
![\vec S \cdot \vec J = \frac{1}{2}(J^2 + S^2 - L^2) = \frac{\hbar^2}{2}[j(j+1) - l(l+1) + s(s+1)]](images/math/f/c/f/fcf4715ea2623ddc3e7d8e67693b9634.png) ,
,
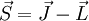 and squaring both sides, we get
and squaring both sides, we get
![\vec L \cdot \vec J = \frac{1}{2}(J^2 - S^2 + L^2) = \frac{\hbar^2}{2}[j(j+1) + l(l+1) - s(s+1)]](images/math/0/9/6/09687161c3ef4f1d289263754ed321c2.png)
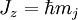 , we obtain the magnetic potential energy of the atom in the applied external magnetic field,
, we obtain the magnetic potential energy of the atom in the applied external magnetic field,
![V_M = \mu_B B m_j \left[ g_L\frac{j(j+1) + l(l+1) - s(s+1)}{2j(j+1)} + g_S\frac{j(j+1) - l(l+1) + s(s+1)}{2j(j+1)} \right]](images/math/a/9/c/a9cb4671032ad22481aca4c5b8eee30f.png) ,
,
 ) and
) and  and
and 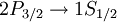 .
.
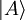 :
:
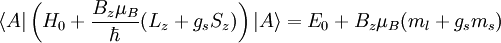 .
.
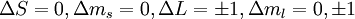 this allows to ignore the spin degree of freedom altogether. As a result, only three spectral lines will be visible, corresponding to the
this allows to ignore the spin degree of freedom altogether. As a result, only three spectral lines will be visible, corresponding to the 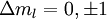 selection rule. The splitting
selection rule. The splitting 

