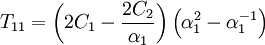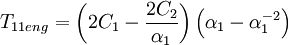To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Mooney-Rivlin solidIn continuum mechanics, a Mooney-Rivlin solid is a generalization of the Neo-Hookean solid model, where the strain energy W is a linear combination of two invariants of Finger tensor
where
where: and C10, C01, and d are constants. (Explanatory gloss for students of applied mathematics, physics, or other disciplines: the characteristic polynomial of the linear operator corresponding to the second rank three-dimensional Finger tensor is usually written In this article, the trace a1 is written I1, the next coefficient a2 is written I2, and the determinant a3 would be written I3.) If The stress tensor The model was proposed by Melvin Mooney and Ronald Rivlin in two independent papers in 1952. Product highlight
Uniaxial elongationNeo-Hookean solid model is an extension of Hooke's law for the case of large deformations. The model of neo-Hookean solid is usable for plastics and rubber-like substances. The response of a neo-Hookean material, or hyperelastic material, to an applied stress differs from that of a linear elastic material. While a linear elastic material has a linear relationship between applied stress and strain, a neo-Hookean material does not. A hyperelastic material will initially be linear, but at a certain point, the stress-strain curve will plateau due to the release of energy as heat while straining the material. Then, at another point, the elastic modulus of the material will increase again. This hyperelasticity, or rubber elasticity, is often observed in polymers. Cross-linked polymers will act in this way because initially the polymer chains can move relative to each other when a stress is applied. However, at a certain point the polymer chains will be stretched to the maximum point that the covalent cross links will allow, and this will cause a dramatic increase in the elastic modulus of the material. One can also use thermodynamics to explain the elasticity of polymers. Neo-Hookean Solid ModelThe model of neo-Hookean solid assumes that the extra stresses due to deformation are proportional to Finger tensor:
where The strain energy for this model is:
where W is potential energy and Usually the model is used for incompressible media. The model was proposed by Ronald Rivlin in 1948. Uni-axial extensionFor the case of uniaxial elongation, true stress can be calculated as: and engineering stress can be calculated as: The Mooney-Rivlin solid model usually fits experimental data better than Neo-Hookean solid does, but requires an additional empirical constant. Brain tissuesElastic response of soft tissues like that in the brain is often modelled based on the Mooney--Rivlin model. Source
Categories: Continuum mechanics | Non-Newtonian fluids | Rubber properties |
|
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Mooney-Rivlin_solid". A list of authors is available in Wikipedia. |


 :
:
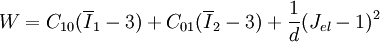 ,
,
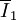 and
and 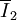 are the first and the second invariant of
are the first and the second invariant of 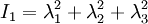 ,
,
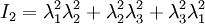 ,
,
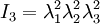 ,
,
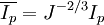 .
.

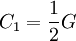 (where G is the
(where G is the  depends upon Finger tensor
depends upon Finger tensor 



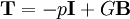 ,
,
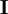 - is the unity tensor, G is a constant equal to
- is the unity tensor, G is a constant equal to 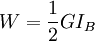 ,
,
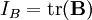 is the trace (or first invariant) of
is the trace (or first invariant) of