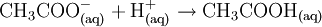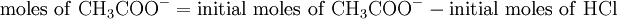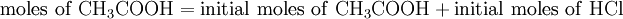To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Buffer solution
Buffer solutions are solutions that resist change in Hydronium ion and the hydroxide ion concentration (and consequently pH) upon addition of small amounts of acid or base, or upon dilution. Buffer solutions consist of a weak acid and its conjugate base (more common) or a weak base and its conjugate acid (less common). The resistive action is the result of the equilibrium between the weak acid (HA) and its conjugate base (A−):
Any alkali added to the solution is consumed by the Hydronium ions. These ions are mostly regenerated as the equilibrium moves to the right and some of the acid dissociates into Hydronium ions and the conjugate base. If a strong acid is added, the conjugate base is protonated, and the pH is almost entirely restored. This is an example of Le Chatelier's principle and the common ion effect. This contrasts with solutions of strong acids or strong bases, where any additional strong acid or base can greatly change the pH. This may be easier to see by comparing two graphs when an strong acid is titrated with a strong base the curve will have a large gradient throughout showing that a small addition of base/acid will have a large effect compared to a weak acid/strong base titration curve which will have a smaller gradient near the pKa. When writing about buffer systems they can be represented as salt of conjugate base/acid, or base/salt of conjugate acid. It should be noted that here buffer solutions are presented in terms of the Brønsted-Lowry notion of acids and bases, as opposed to the Lewis acid-base theory (see acid-base reaction theories). Omitted here are buffer solutions prepared with solvents other than water. Product highlight
Calculating pHThe equilibrium above has the following acid dissociation constant: Simple manipulation with logarithms gives the Henderson-Hasselbalch equation, which describe pH in terms of pKa: In this equation
Maximum buffering capacity is found when pH = pKa, and buffer range is considered to be at a pH = pKa ± 1. Illustration of buffering effect: Sodium acetate/acetic acidThe acid dissociation constant for acetic acid-sodium acetate is given by the equation: Since this equilibrium only involves a weak acid and base, it can be assumed that ionization of the acetic acid and hydrolysis of the acetate ions are negligible. In a buffer consisting of equal amounts of acetic acid and sodium acetate, the equilibrium equation simplifies to
and the pH of the buffer as is equal to the pKa. To determine the effect of addition of a strong acid such as HCl, the following mathematics would provide the new pH. Since HCl is a strong acid, it is completely ionized in solution. This increases the concentration of H+ in solution, which then neutralizes the acetate by the following equation. The consumed hydrogen ions change the effective number of moles of acetic acid and acetate ions: After accounting for volume change to determine concentrations, the new pH could be calculated from the Henderson-Hasselbalch equation. Any neutralization will result in a small change in pH, since it is on a logarithmic scale.. ApplicationsTheir resistance to changes in pH makes buffer solutions very useful for chemical manufacturing and essential for many biochemical processes. The ideal buffer for a particular pH has a pKa equal to the pH desired, since a solution of this buffer would contain equal amounts of acid and base and be in the middle of the range of buffering capacity. Buffer solutions are necessary to keep the correct pH for enzymes in many organisms to work. Many enzymes work only under very precise conditions; if the pH strays too far out of the margin, the enzymes slow or stop working and can denature, thus permanently disabling its catalytic activity. A buffer of carbonic acid (H2CO3) and bicarbonate (HCO3−) is present in blood plasma, to maintain a pH between 7.35 and 7.45. Industrially, buffer solutions are used in fermentation processes and in setting the correct conditions for dyes used in colouring fabrics. They are also used in chemical analysis and calibration of pH meters. Common buffer compounds used in biology
** Values are approximate Making buffer solutionsIn general, preparing a buffer solution requires either:
Both of which in sufficient amounts to maintain the ability to buffer Example: Citric acid-phosphate bufferMake up 0.1M citric acid and 0.2M Disodium hydrogen phosphate solutions then mix as follows to make a 100 ml solution:
See also
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Buffer_solution". A list of authors is available in Wikipedia. |





![\mathrm{K_a = \frac{[H^+][A^-]}{[HA]}}](images/math/a/0/2/a026fcfe3468355857a6b832c680db8c.png)
![pH=pK_a+log_{10}\frac{[A^-]}{[HA]}](images/math/6/7/2/67262074271899856d78d6415b3ae320.png)
![\mathrm{K_a = \frac{[H^+][CH_3COO^-]}{[CH_3COOH]}}](images/math/f/4/d/f4d157b2f1917a0cdc54e7aa18118772.png)