To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Cubic crystal systemThe cubic crystal system (or isometric) is a crystal system where the unit cell is in the shape of a cube. This is one of the most common and simplest shapes found in metallic crystals. Product highlight
Bravais lattices and point/space groupsThe three Bravais lattices which form the cubic crystal system are The simple cubic system consists of one lattice point on each corner of the cube. Each atom at the lattice points is then shared equally between eight adjacent cubes, and the unit cell therefore contains in total one atom (1/8 * 8). The body centered cubic system has one lattice point in the center of the unit cell in addition to the eight corner points. It has a contribution of 2 lattice points per unit cell ((1/8)*8 + 1). Finally, the face centered cubic has lattice points on the faces of the cube of which each unit cube gets exactly one half contribution, in addition to the corner lattice points, giving a total of 4 atoms per unit cell ((1/8 for each corner) * 8 corners + (1/2 for each face) * 6 faces). Attempting to create a C-centered cubic crystal system would result in a simple tetragonal Bravais lattice. There are 8 lattice points on a simple cubic for each corner of the shape. There are 9 lattice points for a body centered because of the extra point in the center of the unit. There are 14 lattice points on a face centered cubic. The point groups and space groups that fall under this crystal system are listed below, using the international notation.
There are 36 cubic space groups, of which 10 are hexoctahedral: Fd3c, Fd3m, Fm3c, Fm3m, Ia3d, Im3m, Pm3m, Pm3n, Pn3m, and Pn3n. Other terms for hexoctahedral are normal class, holohedral, ditesseral central class, galena type. Atomic packing factors and examplesThe cubic crystal system is one of the most common crystal systems found in elemental metals, and naturally occurring crystals and minerals. One very useful way to analyse a crystal is to consider the atomic packing factor. In this approach, the amount of space which is filled by the atoms is calculated under the assumption that they are spherical. Single-element latticesAssuming one atom per lattice point, the atomic packing factor of the simple cubic system is only 0.524. Due to its low density, this is a high energy structure and is rare in nature, but is found in Polonium [1]. Similarly, the body centered structure has a density of 0.680. The higher density makes this a low energy structure which is fairly common in nature. Examples include Fe-iron, Cr-chromium, W-tungsten, and Nb-niobium. Finally, the face centered cubic crystals have a density of 0.741, a ratio that it shares with several other systems, including hexagonal close packed and one version of tetrahedral BCC. This is the most tightly packed crystal possible with spherical atoms. Due to its low energy, FCC is extremely common, examples include lead (for example in lead(II) nitrate), Al-aluminum,Cu- copper,Au- gold and Ag-silver. Multi-element compoundsWhen the compound is formed of two elements whose ions are of roughly the same size, they have what is called the interpenetrating simple cubic structure, where two atoms of a different type have individual simple cubic crystals. However, the unit cell consists of the atom of one being in the middle of the 8 vertices, structurally resembling body centered cubic. The most common example is caesium chloride CsCl. This structure actually has a simple cubic lattice with a two atom basis, the atom positions being atom A at (0,0,0) and and atom B at(0.5,0.5.0.5) However, if the cation is slightly smaller than the anion (a cation/anion radius ratio of 0.414 to 0.732), the crystal forms a different structure, interpenetrating FCC. When drawn separately, both atoms are arranged in an FCC structure. This structure has a FCC lattice, with a two atom basis, the atom positions being atom A at (0,0,0) and atom B at (0.5,0.5,0). The unit cell for this is shown to the left. See also
References
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Cubic_crystal_system". A list of authors is available in Wikipedia. | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||





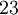
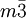
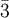
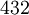

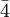 3m
3m