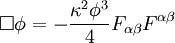Dilaton

In theoretical physics, dilaton originally referred to a theoretical scalar field; as photon refers in one sense to the electromagnetic field. For the dilaton, also known as the radion or graviscalar, it is the scalar field which appears in Kaluza-Klein theory—as the component

g₅₅

Additional recommended knowledge

Better weighing performance in 6 easy steps

Weighing the right way

Correct Test Weight Handling Guide: 12 Practical Tips

of the metric tensor where "5" is the additional circular direction—and obeys an inhomogeneous wave equation, generalizing the Klein-Gordon equation, with extremely strong electromagnetic field as source:

In string theory, also, a dilaton is a particle of a scalar field φ; a scalar field (following the Klein-Gordon equation) that always comes with gravity. Although string theory naturally incorporates Kaluza-Klein theory, perturbative string theories, such as type I string theory, type II string theory and heterotic string theory, already contain the dilaton in the maximal number of 10 dimensions. (On the other hand, M-theory in 11 dimensions does not include dilaton in its spectrum unless it is compactified.)

The exponential of its vacuum expectation value determines the coupling constant g

Therefore the coupling constant is a dynamical variable in string theory, unlike the case of quantum field theory where it is constant. As long as supersymmetry is unbroken, such scalar fields can take arbitrary values (they are moduli). However, supersymmetry breaking usually creates a potential energy for the scalar fields and the scalar fields localize near a minimum whose position should in principle be calculable in string theory.

References

• Y. Fujii, "Mass of the dilaton and the cosmological constant". arXiv:gr-qc/0212030.
• M. Hayashi, T. Watanabe, I. Aizawa and K. Aketo, "Dilatonic Inflation and SUSY Breaking in String-inspired Supergravity". arXiv:hep-ph/0303029.
• F. Alvarenge, A. Batista and J. Fabris, "Does Quantum Cosmology Predict a Constant Dilatonic Field". arXiv:gr-qc/0404034.
• H. Lu, Z. Huang, W. Fang and K. Zhang, "Dark Energy and Dilaton Cosmology". arXiv:hep-th/0409309.
• Paul S. Wesson, Space-Time-Matter, Modern Kaluza-Klein Theory, (1999) World Scientific, Singapore ISBN 981-02-3588-7, p. 31.