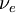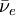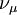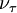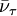To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Neutrino
Neutrinos are elementary particles that travel close to the speed of light, lack an electric charge, are able to pass through ordinary matter almost undisturbed and are thus extremely difficult to detect. As of 2007, it is believed Neutrinos have a minuscule, but non-zero, mass too small to be measured. They are usually denoted by the Greek letter ν (nu). Neutrinos are created as a result of certain types of radioactive decay or nuclear reactions such as those that take place in the sun, in nuclear reactors, or when cosmic rays hit atoms. There are three types, or "flavors", of neutrinos: electron neutrinos, muon neutrinos and tau neutrinos; each type also has an antimatter partner, called an antineutrino. Electron neutrinos are generated whenever protons change into neutrons, while electron antineutrinos are generated whenever neutrons change into protons. These are the two forms of beta decay. Interactions involving neutrinos are generally mediated by the weak nuclear force. Most neutrinos passing through the Earth emanate from the sun, and more than 50 trillion solar electron neutrinos pass through the human body every second. Product highlight
HistoryThe neutrino was first postulated in December 1930 by Wolfgang Pauli to explain conservation of energy in beta decay, the decay of a neutron into a proton and an electron. Pauli theorized that an undetected particle was carrying away the observed difference between the energy and momentum of the initial and final particles. In 1942 Kan-Chang Wang first proposed to use beta-capture to experimentally detect neutrinos.[1] In 1956 Clyde Cowan, Frederick Reines, F. B. Harrison, H. W. Kruse, and A. D. McGuire published the article "Detection of the Free Neutrino: a Confirmation" in Science, a result that was rewarded with the 1995 Nobel Prize. In this experiment, now known as the neutrino experiment, neutrinos created in a nuclear reactor by beta decay were shot into protons producing neutrons and positrons both of which could be detected. It is now known that both the proposed and the observed particles were antineutrinos. The name neutrino was coined by Enrico Fermi, who developed the first theory describing neutrino interactions, as a pun on neutrone, the Italian name of the neutron: neutrone seems to use the -one suffix (even if it is a complete word, not a compound), which in Italian indicates a large object, whereas -ino indicates a small one. In 1962 Leon M. Lederman, Melvin Schwartz and Jack Steinberger showed that more than one type of neutrino exists by first detecting interactions of the muon neutrino, which earned them the 1988 Nobel Prize. When a third type of lepton, the tau, was discovered in 1975 at the Stanford Linear Accelerator, it too was expected to have an associated neutrino. First evidence for this third neutrino type came from the observation of missing energy and momentum in tau decays analogous to the beta decay leading to the discovery of the neutrino. The first detection of tau neutrino interactions was announced in summer of 2000 by the DONUT collaboration at Fermilab, making it the latest particle of the Standard Model, whose existence was already inferred both by theoretical consistency, as well as experimental data from LEP to have been directly observed. Starting in the late 1960s, several experiments found that the number of electron neutrinos arriving from the sun was between one third and one half the number predicted by the Standard Solar Model, a discrepancy which became known as the solar neutrino problem and remained unresolved for some thirty years. The Standard Model of particle physics assumes massless neutrinos and conserves flavors. However, non-zero neutrino mass and accompanying flavor oscillation remained a possibility. A practical method for investigating neutrino masses (that is, flavor oscillation) was first suggested by Bruno Pontecorvo in 1957 using an analogy with the neutral kaon system; over the subsequent 10 years he developed the mathematical formalism and the modern formulation of vacuum oscillations. In 1985 Stanislav Mikheyev and Alexei Smirnov (expanding on 1978 work by Lincoln Wolfenstein) noted that flavor oscillations can be modified when neutrinos propagate through matter. This so-called MSW effect is important to understand neutrinos emitted by the Sun, which pass through its dense atmosphere on their way to detectors on Earth. Starting in 1998, experiments began to show that solar and atmospheric neutrinos change flavors (see Super-Kamiokande, Sudbury Neutrino Observatory). Although individual experiments, such as the set of solar neutrino experiments, are consistent with non-oscillatory mechanisms of neutrino flavor conversion, taken altogether, neutrino experiments imply the existence of neutrino oscillations. Especially relevant in this context are the reactor experiment KamLAND and the accelerator experiments such as MINOS. The KamLAND experiment has indeed identified oscillations as the neutrino flavor conversion mechanism involved in the resolution of the solar neutrino problem: the electron neutrinos produced in the sun had partly changed into other flavors which the experiments could not detect. Similarly MINOS confirms the oscillation of atmospheric neutrinos and gives a better determination of the mass squared splitting (Maltoni, 2004). Raymond Davis Jr. and Masatoshi Koshiba were jointly awarded the 2002 Nobel Prize in Physics. Ray Davis for his pioneer work on solar neutrinos and Koshiba for the first real time observation of supernova neutrinos. The detection of solar neutrinos, and of neutrinos of SN 1987A supernova in 1987 marked the beginning of neutrino astronomy. PropertiesThe neutrino has half-integer spin ( Because the cross section in weak nuclear interactions is very small, neutrinos can pass through matter almost unhindered. For typical neutrinos produced in the sun (with energies of a few MeV), it would take approximately one light year (~1016m) of lead to block half of them. Detection of neutrinos is therefore challenging, requiring large detection volumes or high intensity artificial neutrino beams. All neutrinos observed to date have left-handed chirality. Types of neutrinos
There are three known types (flavours) of neutrinos: electron neutrino νe, muon neutrino νμ and tau neutrino ντ, named after their partner leptons in the Standard Model (see table at right). The current best measurement of the number of neutrino types comes from observing the decay of the Z boson. This particle can decay into any light neutrino and its antineutrino, and the more types of light neutrinos available, the shorter the lifetime of the Z boson. Measurements of the Z lifetime have shown that the number of light neutrino types (with "light" meaning of less than half the Z mass) is 3.[3] The correspondence between the six quarks in the Standard Model and the six leptons, among them the three neutrinos, suggests to physicists' intuition that there should be exactly three types of neutrino. However, actual proof that there are only three kinds of neutrinos remains an elusive goal of particle physics. The possibility of sterile neutrinos — relatively light neutrinos which do not participate in the weak interaction but which could be created through flavour oscillation (see below) — is unaffected by these Z-boson-based measurements, and the existence of such particles is in fact hinted by experimental data from the LSND experiment. However the currently running MiniBooNE experiment suggested, until recently, that sterile neutrinos are not required to explain the experimental data[4], although the latest research into this area is on-going and anomalies in the MiniBooNE data may allow for exotic neutrino types, including sterile neutrinos.[5] Flavour OscillationsNeutrinos are most often created or detected with a well defined flavour (electron, muon, tau). However, in a phenomenon known as neutrino flavour oscillation, neutrinos are able to oscillate between the three available flavors while they propagate through space. Specifically, this occurs because the neutrino flavor eigenstates are not the same as the neutrino mass eigenstates (simply called 1, 2, 3). This allows for a neutrino that was produced as an electron neutrino at a given location to have a calculable probability to be detected as either a muon or tau neutrino after it has traveled to another location. This quantum mechanical effect was first hinted by the discrepancy between the number of electron neutrinos detected from the sun's core failing to match the expected numbers, dubbed as the "solar neutrino problem". In the Standard Model the existence of flavor oscillations implies a non-zero neutrino mass, because the amount of mixing between neutrino flavors at a given time depends on the differences in their squared-masses (although it is not generally so, on the Standard Model mixing would be zero for massless neutrinos). In keeping with their massive nature, it is still possible that the neutrino and antineutrino are in fact the same particle, a hypothesis first proposed by the Italian physicist Ettore Majorana. The reason for the need for mass to make neutrinos equivalent to antineutrinos, is that only with a massive particle (which therefore cannot move at the speed of light) is it possible to postulate an inertial frame which moves faster than the particle, and thereby converts its spin from one type of "handedness" to the other (for example, right to left-handed spin), thus making any type of neutrino in the new frame, appear as its own antiparticle. MassThe Standard Model of particle physics assumes that neutrinos are massless, although adding massive neutrinos to the basic framework is not difficult. Indeed, the experimentally established phenomenon of neutrino oscillation requires neutrinos to have non-zero masses.[4] The strongest upper limit on the masses of neutrinos comes from cosmology: the Big Bang model predicts that there is a fixed ratio between the number of neutrinos and the number of photons in the cosmic microwave background. If the total energy of all three types of neutrinos exceeded an average of 50 electronvolts per neutrino, there would be so much mass in the universe that it would collapse. This limit can be circumvented by assuming that the neutrino is unstable; however, there are limits within the Standard Model that make this difficult. A much more stringent constraint comes from a careful analysis of cosmological data, such as the cosmic microwave background radiation, galaxy surveys and the Lyman-alpha forest. These indicate that the sum of the neutrino masses must be less than 0.3 electronvolt (Goobar, 2006). In 1998, research results at the Super-Kamiokande neutrino detector determined that neutrinos do indeed flavour oscillate, and therefore have mass. The experiment is only sensitive to the difference in the squares of the masses. These differences are known to be very small, about 0.05 electronvolt (Mohapatra, 2005). The best estimate of the difference in the squares of the masses of mass eigenstates 1 and 2 was published by KamLAND in 2005: Δm212 = 0.000079 eV2 In 2006, the MINOS experiment measured oscillations from an intense muon neutrino beam, determining the difference in the squares of the masses between neutrino mass eigenstates 2 and 3. The initial results indicate Δm232 = 0.0031 eV2, consistent with previous results from Super-K.[6] Currently a number of efforts are under way to directly determine the absolute neutrino mass scale in laboratory experiments. The methods applied involve nuclear beta decay (KATRIN and MARE) or neutrinoless double beta decay (e.g. GERDA, CUORE/Cuoricino, NEMO 3 and others). HandednessExperimental results show that (nearly) all produced and observed neutrinos have left-handed helicities (spins antiparallel to momenta), and all antineutrinos have right-handed helicities, within the margin of error. In the massless limit, it means that only one of two possible chiralities is observed for either particle. These are the only chiralities included in the Standard Model of particle interactions. It is possible that their counterparts (right-handed neutrinos and left-handed antineutrinos) simply do not exist. If they do, their properties are substantially different from observable neutrinos and antineutrinos. It is theorized that they are either very heavy (on the order of GUT scale — see Seesaw mechanism), do not participate in weak interaction (so-called sterile neutrinos), or both. The existence of nonzero neutrino masses somewhat complicates the situation. Neutrinos are produced in weak interactions as chirality eigenstates. However, chirality of a massive particle is not a constant of motion; helicity is, but the chirality operator does not share eigenstates with the helicity operator. Free neutrinos propagate as mixtures of left- and right-handed helicity states, with mixing amplitudes on the order of mν / E. This does not significantly affect the experiments, because neutrinos involved are nearly always ultrarelativistic, and thus mixing amplitudes are vanishingly small (for example, most solar neutrinos have energies on the order of 100 keV–1 MeV, so the fraction of neutrinos with "wrong" helicity among them can't exceed 10 − 10). [7][8] Neutrino sourcesArtificially produced neutrinosNuclear reactors are the major source of human-generated neutrinos. Anti-neutrinos are made in the beta-decay of neutron-rich daughter fragments in the fission process. Generally, the four main isotopes contributing to the anti-neutrino flux are: uranium-235, uranium-238, plutonium-239 and plutonium-241. An average nuclear power plant may generate over 1020 anti-neutrinos per second. Some particle accelerators have been used to make neutrino beams. The technique is to smash protons into a fixed target, producing charged pions or kaons. These unstable particles are then magnetically focused into a long tunnel where they decay while in flight. Because of the relativistic boost of the decaying particle the neutrinos are produced as a beam rather than isotropically. Nuclear bombs also produce very large numbers of neutrinos. Fred Reines and Clyde Cowan thought about trying to detect neutrinos from a bomb before they switched to looking for reactor neutrinos. Geologically produced neutrinosNeutrinos are produced as a result of natural background radiation. In particular, the decay chains of uranium-238 and thorium-232 isotopes, as well as potassium-40, include beta decays which emit anti-neutrinos. These so-called geoneutrinos can provide valuable information on the Earth's interior. A first indication for geoneutrinos was found by the KamLAND experiment in 2005. KamLAND's main background in the geoneutrino measurement are the anti-neutrinos coming from reactors. Several future experiments aim at improving the geoneutrino measurement and these will necessarily have to be far away from reactors. Atmospheric neutrinosAtmospheric neutrinos result from the interaction of cosmic rays with atomic nuclei in the Earth's atmosphere, creating showers of particles, many of which are unstable and produce neutrinos when they decay. A collaboration of particle physicists from Tata Institute of Fundamental Research (TIFR), India, Osaka City University, Japan and Durham University, UK recorded the first cosmic ray neutrino interaction in an underground laboratory in KGF gold mines in India in 1965. Solar neutrinosSolar neutrinos originate from the nuclear fusion powering the sun and other stars. The details of the operation of the sun are explained by the Standard Solar Model. In short: when four protons fuse to become one helium nucleus, two of them have to convert into neutrons, and each such conversion releases one electron neutrino. The sun sends enormous numbers of neutrinos in all directions. Every second, about 70 billion (7×1010) solar neutrinos pass through every square centimeter on Earth that faces the sun.[9] Since neutrinos are insignificantly absorbed by the mass of the Earth, the surface area on the side of the Earth opposite the Sun receives about the same number of neutrinos as the side facing the Sun. SupernovaeNeutrinos are an important product of Types Ib, Ic and II (core-collapse) supernovae. In such events, the pressure at the core becomes so high (1014 g/cm³) that the degeneracy of electrons is not enough to prevent protons and electrons from combining to form a neutron and an electron neutrino. A second and more important neutrino source is the thermal energy (100 billion Kelvin) of the newly formed neutron core, which is dissipated via the formation of neutrino-antineutrino pairs of all flavors.[10] Most of the energy produced in supernovas is thus radiated away in the form of an immense burst of neutrinos. The first experimental evidence of this phenomenon came in the year 1987, when neutrinos from supernova 1987A were detected. The water-based detectors Kamiokande II and IMB detected 11 and 8 antineutrinos of thermal origin,[10] respectively, while the gallium-71-based Baksan detector found 5 neutrinos (lepton number = 1) of either thermal or electron-capture origin, in a burst lasting less than 13 seconds. It is thought that neutrinos would also be produced from other events such as the collision of neutron stars. Because neutrinos interact so little with matter, it is thought that a supernova's neutrino emissions carry information about the innermost regions of the explosion. Much of the visible light comes from the decay of radioactive elements produced by the supernova shock wave, and even light from the explosion itself is scattered by dense and turbulent gases. Neutrinos, on the other hand, pass through these gases, providing information about the supernova core (where the densities were large enough to influence the neutrino signal). Furthermore, the neutrino burst is expected to reach Earth before any electromagnetic waves, including visible light, gamma rays or radio waves. The exact time delay is unknown, but for a Type II supernova, astronomers expect the neutrino flood to be released seconds after the stellar core collapse, while the first electromagnetic signal may be hours or days later. The SNEWS project uses a network of neutrino detectors to monitor the sky for candidate supernova events; it is hoped that the neutrino signal will provide a useful advance warning of an exploding star. The energy of supernova neutrinos ranges from a few to several tens of MeV. However, the sites where cosmic rays are accelerated are expected to produce neutrinos that are one million times more energetic or more, produced from turbulent gasesous environments left over by supernova explosions: the supernova remnants. The connection between cosmic rays and supernova remnants was suggested by Walter Baade and Fritz Zwicky, shown to be consistent with the cosmic ray losses of the Milky Way if the efficiency of acceleration is about 10 percent by Ginzburg and Syrovatsky, and it is supported by a specific mechanism called "shock wave acceleration" based on Fermi ideas (which is still under development). The very high energy neutrinos are still to be seen, but this branch of neutrino astronomy is just in its infancy. The main existing or forthcoming experiments that aim at observing very high energy neutrinos from our galaxy are Baikal, AMANDA, ICECUBE, Antares, NEMO and Nestor. Related information is provided by very high energy gamma ray observatories, such as HESS and MAGIC. Indeed, the collisions of cosmic rays are supposed to produce charged pions, whose decay give the neutrinos, but also neutral pions, whose decay give gamma rays: the environment of a supernova remnant is transparent to both types of radiation. Still higher energy neutrinos, resulting from the interactions of extragalactic cosmic rays, could be observed with the cosmic ray observatory Auger or with the dedicated experiment named ANITA. Cosmic background radiationIt is thought that, just like the cosmic microwave background radiation left over from the Big Bang, there is a background of low energy neutrinos in our Universe. In the 1980s it was proposed that these may be the explanation for the dark matter thought to exist in the universe. Neutrinos have one important advantage over most other dark matter candidates: we know they exist. However, they also have serious problems. From particle experiments, it is known that neutrinos are very light. This means that they move at speeds close to the speed of light except when they have extremely low kinetic energy. Thus, dark matter made from neutrinos is termed "hot dark matter". The problem is that being fast moving, the neutrinos would tend to have spread out evenly in the universe before cosmological expansion made them cold enough to congregate in clumps. This would cause the part of dark matter made of neutrinos to be smeared out and unable to cause the large galactic structures that we see. Further, these same galaxies and groups of galaxies appear to be surrounded by dark matter which is not fast enough to escape from those galaxies. Presumably this matter provided the gravitational nucleus for formation. This implies that neutrinos make up only a small part of the total amount of dark matter. From cosmological arguments, relic background neutrinos are estimated to have density of 56 of each type per cubic centimeter and temperature 1.9K (1.7×10-4eV) if they are massless, much colder if their mass exceeds 0.001 eV. Although their density is quite high, due to extremely low neutrino cross-sections at sub-eV energies, the relic neutrino background has not yet been observed in the laboratory (e.g. (boron-8 solar neutrinos -- which are emitted with a higher energy -- have been detected definitively despite having a space density that is lower than that of relic neutrinos by some 6 orders of magnitude). Neutrino detectionNeutrinos can interact via the neutral current (involving the exchange of a Z boson) or charged current (involving the exchange of a W boson) weak interactions.
Antineutrinos were first detected in 1953 near a nuclear reactor. Reines and Cowan used two targets containing a solution of cadmium chloride in water. Two scintillation detectors were placed next to the cadmium targets. Antineutrino charged current interactions with the protons in the water produced positrons and neutrons. The resulting positron annihilations with electrons created photons with an energy of about 0.5 MeV. Pairs of photons in coincidence could be detected by the two scintillation detectors above and below the target. The neutrons were captured by cadmium nuclei resulting in gamma rays of about 8 MeV that were detected a few microseconds after the photons from a positron annihilation event. Today, the much larger KamLAND detector uses similar techniques and 53 Japanese nuclear power plants to study neutrino oscillation. Chlorine detectors consist of a tank filled with a chlorine containing fluid such as Tetrachloroethylene. A neutrino converts a chlorine atom into one of argon via the charged current interaction. The fluid is periodically purged with helium gas which would remove the argon. The helium is then cooled to separate out the argon. A chlorine detector in the former Homestake Mine near Lead, South Dakota, containing 520 short tons (470 metric tons) of fluid, made the first measurement of the deficit of electron neutrinos from the sun (see solar neutrino problem). A similar detector design uses a gallium → germanium transformation which is sensitive to lower energy neutrinos. This latter method is nicknamed the "Alsace-Lorraine" technique because of the reaction sequence (gallium-germanium-gallium) involved. These chemical detection methods are useful only for counting neutrinos; no neutrino direction or energy information is available. "Ring-imaging" detectors take advantage of the Cherenkov light produced by charged particles moving through a medium faster than the speed of light in that medium. In these detectors, a large volume of clear material (e.g., water) is surrounded by light-sensitive photomultiplier tubes. A charged lepton produced with sufficient energy creates Cherenkov light which leaves a characteristic ring-like pattern of activity on the array of photomultiplier tubes. This pattern can be used to infer direction, energy, and (sometimes) flavor information about the incident neutrino. Two water-filled detectors of this type (Kamiokande and IMB) recorded the neutrino burst from supernova 1987a. The largest such detector is the water-filled Super-Kamiokande. The Sudbury Neutrino Observatory (SNO) uses heavy water. In addition to the neutrino interactions available in a regular water detector, the deuterium in the heavy water can be broken up by a neutrino. The resulting free neutron is subsequently captured, releasing a burst of gamma rays which are detected. All three neutrino flavors participate equally in this dissociation reaction. The MiniBooNE detector employs pure mineral oil as its detection medium. Mineral oil is a natural scintillator, so charged particles without sufficient energy to produce Cherenkov light can still produce scintillation light. This allows low energy muons and protons, invisible in water, to be detected. Tracking calorimeters such as the MINOS detectors use alternating planes of absorber material and detector material. The absorber planes provide detector mass while the detector planes provide the tracking information. Steel is a popular absorber choice, being relatively dense and inexpensive and having the advantage that it can be magnetised. The NOνA proposal suggests eliminating the absorber planes in favor of using a very large active detector volume. The active detector is often liquid or plastic scintillator, read out with photomultiplier tubes, although various kinds of ionisation chambers have also been used. Tracking calorimeters are only useful for high energy (GeV range) neutrinos. At these energies, neutral current interactions appear as a shower of hadronic debris and charged current interactions are identified by the presence of the charged lepton's track (possibly alongside some form of hadronic debris.) A muon produced in a charged current interaction leaves a long penetrating track and is easy to spot. The length of this muon track and its curvature in the magnetic field provide energy and charge (μ + versus μ − ) information. An electron in the detector produces an electromagnetic shower which can be distinguished from hadronic showers if the granularity of the active detector is small compared to the physical extent of the shower. Tau leptons decay essentially immediately to either pions or another charged lepton, and can't be observed directly in this kind of detector. (To directly observe taus, one typically looks for a kink in tracks in photographic emulsion.) Most neutrino experiments must address the flux of cosmic rays that bombard the earth's surface. The higher energy (>50 MeV or so) neutrino experiments often cover or surround the primary detector with a "veto" detector which reveals when a cosmic ray passes into the primary detector, allowing the corresponding activity in the primary detector to be ignored ("vetoed"). For lower energy experiments, the cosmic rays are not directly the problem. Instead, the spallation neutrons and radioisotopes produced by the cosmic rays may mimic the desired physics signals. For these experiments, the solution is to locate the detector deep underground so that the earth above can reduce the cosmic ray rate to tolerable levels. Neutrino experiments, neutrino detectorsGeneral data
Technical data
Notation Sensitivity (1)
Sensitivity (2)
Type of process
Research Institution
Motivation for scientific interest in the neutrinoThe neutrino is of scientific interest because it can make an exceptional probe for environments that are typically concealed from the standpoint of other observation techniques, such as optical and radio observation. The first such use of neutrinos was proposed in the early 20th century for observation of the core of the Sun. Direct optical observation of the solar core is impossible due to the diffusion of electromagnetic radiation by the huge amount of matter surrounding the core. On the other hand, neutrinos generated in stellar fusion reactions are very weakly interacting and therefore pass right through the sun with few or no interactions. While photons emitted by the solar core may require 1,000 years to diffuse to the outer layers of the Sun, neutrinos are virtually unimpeded and cross this distance at nearly the speed of light. Neutrinos are also useful for probing astrophysical sources beyond our solar system. Neutrinos are the only known particles that are not significantly attenuated by their travel through the interstellar medium. Optical photons can be obscured or diffused by dust, gas and background radiation. High-energy cosmic rays, in the form of fast-moving protons and atomic nuclei, are not able to travel more than about 100 megaparsecs due to the GZK cutoff. Neutrinos can travel this distance, and greater distances, with very little attenuation. The galactic core of the Milky Way is completely obscured by dense gas and numerous bright objects. However, it is likely that neutrinos produced in the galactic core will be measurable by Earth-based neutrino telescopes in the next decade. The most important use of the neutrino is in the observation of supernovae, the explosions that end the lives of highly massive stars. The core collapse phase of a supernova is an almost unimaginably dense and energetic event. It is so dense that no known particles are able to escape the advancing core front except for neutrinos. Consequently, supernovae are known to release approximately 99% of their energy in a rapid (10 second) burst of neutrinos. As a result, the usefulness of neutrinos as a probe for this important event in the death of a star cannot be overstated. Determining the mass of the neutrino (see above) is also an important test of cosmology (see Dark matter). Many other important uses of the neutrino may be imagined in the future. It is clear that the astrophysical significance of the neutrino as an observational technique is comparable with all other known techniques, and is therefore a major focus of study in astrophysical communities. In particle physics the main virtue of studying neutrinos is that they are typically the lowest mass, and hence lowest energy examples of particles theorized in extensions of the Standard Model of particle physics. For example, one would expect that if there is a fourth class of fermions beyond the electron, muon, and tau generations of particles, that a fourth generation neutrino would be the easiest to generate in a particle accelerator. Neutrinos could also be used for studying quantum gravity effects. Because they are not affected by either the strong interaction or electromagnetism, and because they are not normally found in composite particles (unlike quarks) or prone to near instantaneous decay (like many other standard model particles) it might be possible to isolate and measure gravitational effects on neutrinos at a quantum level. See also
neutrino physicists
Notes
References
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Neutrino". A list of authors is available in Wikipedia. | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||





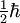 ) and is therefore a
) and is therefore a