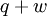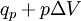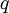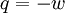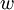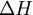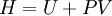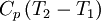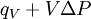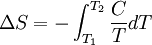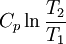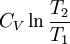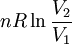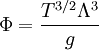To use all functions of this page, please activate cookies in your browser.
my.chemeurope.com
With an accout for my.chemeurope.com you can always see everything at a glance – and you can configure your own website and individual newsletter.
- My watch list
- My saved searches
- My saved topics
- My newsletter
Ideal gasAn ideal gas or perfect gas is a hypothetical gas consisting of identical particles of zero volume, with no intermolecular forces. Additionally, the constituent atoms or molecules undergo perfectly elastic collisions with the walls of the container. Real gases do not exhibit these exact properties, although the approximation is often good enough to describe real gases. Product highlight
Types of ideal gasesThere exist three basic types of ideal gas:
The classical ideal gas can be separated into two types: The classical thermodynamic ideal gas and the ideal quantum Boltzmann gas. Both are essentially the same, except that the classical thermodyamic ideal gas is based on classical thermodynamics alone, and certain thermodynamic parameters such as the entropy are only specified to within an undetermined additive constant. The ideal quantum Boltzmann gas overcomes this limitation by taking the limit of the quantum Bose gas and quantum Fermi gas in the limit of high temperature to specify these additive constants. The behavior of a quantum Boltzmann gas is the same as that of a classical ideal gas except for the specification of these constants. The results of the quantum Boltzmann gas are used in a number of cases including the Sackur-Tetrode equation for the entropy of an ideal gas and the Saha ionization equation for a weakly ionized plasma. Classical thermodynamic ideal gasThe thermodynamic properties of an ideal gas can be described by two equations: The equation of state of a classical ideal gas is given by the ideal gas law. The internal energy of an ideal gas is given by: where:
The probability distribution of particles by velocity or energy is given by the Boltzmann distribution. The ideal gas law is an extension of experimentally discovered gas laws. Real fluids at low density and high temperature approximate the behavior of a classical ideal gas. However, at lower temperatures or a higher density, a real fluid deviates strongly from the behavior of an ideal gas, particularly as it condenses from a gas into a liquid or solid. Heat capacityThe heat capacity at constant volume of an ideal gas is: It is seen that the constant The heat capacity at constant pressure of an ideal gas is: where H = U + pV is the enthalpy of the gas. It is seen that EntropyUsing the results of thermodynamics only, we can go a long way in determining the expression for the entropy of an ideal gas. This is an important step since, according to the theory of thermodynamic potentials, of which the internal energy U is one, if we can express the entropy as a function of U and the volume V, then we will have a complete statement of the thermodynamic behavior of the ideal gas. We will be able to derive both the ideal gas law and the expression for internal energy from it. Since the entropy is an exact differential, using the chain rule, the change in entropy when going from a reference state 0 to some other state with entropy S may be written as ΔS where: where the reference variables may be functions of the number of particles N. Using the definition of the heat capacity at constant volume for the first differential and the appropriate Maxwell relation for the second we have: Expressing CV in terms of where all constants have been incorporated into the logarithm as f(N) which is some function of the particle number N having the same dimensions as From this we find an equation for the function f(N) Differentiating this with respect to a, setting a equal to unity, and then solving the differential equation yields f(N): where φ is some constant with the dimensions of This is about as far as we can go using thermodynamics alone. Note that the above equation is flawed — as the temperature approaches zero, the entropy approaches negative infinity, in contradiction to the third law of thermodynamics. In the above "ideal" development, there is a critical point, not at absolute zero, at which the argument of the logarithm becomes unity, and the entropy becomes zero. This is unphysical. The above equation is a good approximation only when the argument of the logarithm is much larger than unity — the concept of an ideal gas breaks down at low values of V/N. Nevertheless, there will be a "best" value of the constant in the sense that the predicted entropy is as close as possible to the actual entropy, given the flawed assumption of ideality. It remained for quantum mechanics to introduce a reasonable value for the value of φ which yields the Sackur-Tetrode equation for the entropy of an ideal gas. It too suffers from a divergent entropy at absolute zero, but is a good approximation to an ideal gas over a large range of densities. Thermodynamic potentialsSince the dimensionless heat capacity at constant pressure where Φ is now the undetermined constant. The chemical potential of the ideal gas is calculated from the corresponding equation of state (see thermodynamic potential): where G is the Gibbs free energy and is equal to U + pV − TS so that: The thermodynamic potentials for an ideal gas can now be written as functions of T, V, and N as: The most informative way of writing the potentials is in terms of their natural variables, since each of these equations can be used to derive all of the other thermodynamic variables of the system. In terms of their natural variables, the thermodynamic potentials of a single-specie ideal gas are: In statistical mechanics, the relationship between the Helmholtz free energy and the partition function is fundamental, and is used to calculate the thermodynamic properties of matters; see configuration integral for more details.
Multicomponent systemsBy Gibbs theorem, the entropy of a multicomponent system is equal to the sum of the entropies of each chemical species (assuming no surface effects). The entropy of a multicomponent system will be: where the sum is over all species. Likewise, the free energies are equal to the sums of the free energies of each species so that if Φ is a thermodynamic potential then where Φj is expressed in terms of its natural variables. For example, the internal energy will be: where N is defined as
Speed of soundThe speed of sound in an ideal gas is given by where
Equation Table for an Ideal Gas (pVm = constant)The following table gives the values for the change in the value of some thermodynamic variables under the specified transformation.
Ideal quantum gasesIn the above mentioned Sackur-Tetrode equation, the best choice of the entropy constant was found to be proportional to the quantum thermal wavelength of a particle, and the point at which the argument of the logarithm becomes zero is roughly equal to the point at which the average distance between particles becomes equal to the thermal wavelength. In fact, quantum theory itself predicts the same thing. Any gas behaves as an ideal gas at high enough temperature and low enough density, but at the point where the Sackur-Tetrode equation begins to break down, the gas will begin to behave as a quantum gas, composed of either bosons or fermions. (See the gas in a box article for a derivation of the ideal quantum gases, including the ideal Boltzmann gas.) Ideal Boltzmann gasThe ideal Boltzmann gas yields the same results as the classical thermodynamic gas, but makes the following identification for the undetermined constant Φ: where Λ is the thermal de Broglie wavelength of the gas and g is the degeneracy of states. Ideal Bose and Fermi gasesAn ideal gas of bosons (e.g. a photon gas) will be governed by Bose-Einstein statistics and the distribution of energy will be in the form of a Bose-Einstein distribution. An ideal gas of fermions will be governed by Fermi-Dirac statistics and the distribution of energy will be in the form of a Fermi-Dirac distribution. See also
|
||||||||||||||||||||||||||||||||||||||||||||||||
| This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Ideal_gas". A list of authors is available in Wikipedia. |





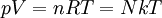
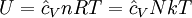
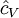 is a constant (e.g. equal to 3/2 for a monatomic gas)
is a constant (e.g. equal to 3/2 for a monatomic gas)
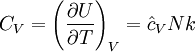
 while for a diatomic gas it is
while for a diatomic gas it is 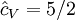 . It is seen that macroscopic measurements on heat capacity provide information on the microscopic structure of the molecules.
. It is seen that macroscopic measurements on heat capacity provide information on the microscopic structure of the molecules.
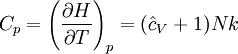
 is also a constant and that the dimensionless heat capacities are related by:
is also a constant and that the dimensionless heat capacities are related by:
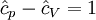
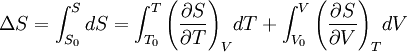

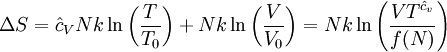
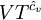 in order that the argument of the logarithm be dimensionless. We now impose the constraint that the entropy be extensive. This will mean that when the extensive parameters (V and N) are multiplied by a constant, the entropy will be multiplied by the same constant. Mathematically:
in order that the argument of the logarithm be dimensionless. We now impose the constraint that the entropy be extensive. This will mean that when the extensive parameters (V and N) are multiplied by a constant, the entropy will be multiplied by the same constant. Mathematically:
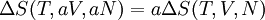
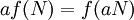
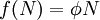
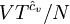 . Substituting into the equation for the change in entropy:
. Substituting into the equation for the change in entropy:

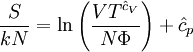

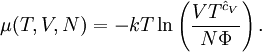

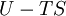

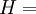
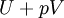
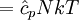
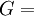
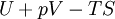
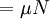

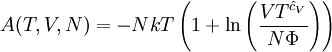
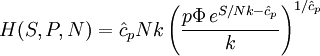
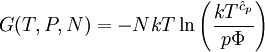
![S=\sum_j S_j = \sum_j N_j\,k \left[\ln\left( \frac{VT^{\hat{c}_V}}{N_j\Phi}\right)+\hat{c}_p\right]](images/math/5/a/d/5ad6e0c2254681cc73ac172c33f60f7f.png)
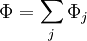
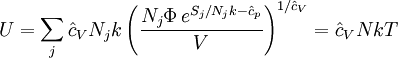
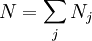 .
.
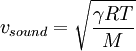
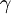 is the
is the 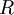 is the universal gas constant
is the universal gas constant
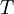 is the
is the 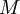 is the
is the 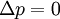
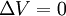
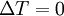

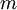
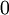

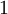
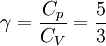
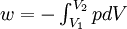

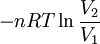
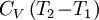
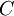
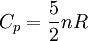
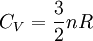
 or
or 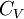
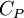 or
or