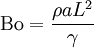My watch list

Bond number

In fluid mechanics, the Bond number, notated Bo, is a dimensionless number expressing the ratio of body forces (often gravitational) to surface tension forces:

${\rm Bo} = \frac{\rho a L^2}{\gamma}$

Additional recommended knowledge

Recognize and detect the effects of electrostatic charges on your balance

Essential Laboratory Skills Guide

Weighing the right way

where

$ρ$ is the density, or the density difference between fluids.
$a$ the acceleration associated with the body force, almost always gravity.
$L$ the 'characteristic length scale', e.g. radius of a drop or the radius of a capillary tube.
$γ$ is the surface tension of the interface.

Sometimes the density scale used is the difference in density between the two phases, $Δρ$ .

The Bond number is a measure of the importance of surface tension forces compared to body forces. A high Bond number indicates that the system is relatively unaffected by surface tension effects; a low number (typically less than one is the requirement) indicates that surface tension dominates. Intermediate numbers indicate a non-trivial balance between the two effects.

The Bond number is the most common comparison of gravity and surface tension effects and it may be derived in a number of ways, such as scaling the pressure of a drop of liquid on a solid surface. It is usually important, however, to find the right length scale specific to a problem by doing a ground-up scale analysis. Other dimensionless numbers are related to the Bond number:

$\rm Bo = Eo = 2 Go^2 = 2 De^2\,$

Where $Eo,Go,$ and $De$ are respectively the Eötvös, Goucher, and Deryagin numbers. The "difference" between the Goucher and Deryagin numbers is that the Goucher number (arises in wire coating problems) uses the letter $R$ to represent length scales while the Deryagin number (arises in plate film thickness problems) uses $L$ .